1. Introduction
Within the last decades, adhesive bonding became a very common assembly technique in many industrial sectors, such as aeronautical (e.g., in composite aircraft to bond the stringers to fuselage and wing skins to stiffen the structures against buckling [
1]), civil (e.g., in glass-fiber-reinforced polymer pultruded beams [
2] or in carbon-fiber-reinforced polymer beams [
3]), automotive (e.g., in both closures and structural modules [
4]), and biomedical engineering (to fix implants in bone tissue in orthopedic or dentistry surgery [
5]), as an alternative to conventional joining techniques, such as welding and riveting [
6]. Adhesive bonding provides several advantages, including reduced stress concentrations, higher corrosion resistance, water tightness, and the ability to join materials with dissimilar properties. Moreover, this technique is increasingly chosen by the transport industry (automotive and aeronautics) because it allows the production of lighter structures, thus reducing
emissions. Nevertheless, adhesive bonding still presents some disadvantages. One of the main concerns limiting the use of adhesive joints is their long-life durability when exposed to service conditions [
4]. Corrosion and aging may cause micro-cracking phenomena that can be measured via non-destructive techniques [
7]. Another drawback is represented by the multifactorial and multiscale nature of the damage phenomena occurring in the adhesive joints, which make it more complex to predict their strength.
In some structural polymeric adhesives, the tensile stress–strain behavior is typically characterized by an initial linear-elastic phase, followed by softening and rupture. This nonlinear constitutive behavior suggests that a micro-cracking process could occur: pre-existing microcracks, generated by the adhesive preparation (manufacturing, thermal treatment, etc.) and initially present in the linear-elastic phase, propagate during the softening phase, causing debonding and failure [
8].
Generally, tube-to-tube butt joints are used to experimentally characterize the mechanical properties of structural adhesives under combined tensile–torsion loads [
9,
10,
11]. Despite numerous experimental studies on this subject, it is still not possible to univocally define the damage/failure behavior of adhesive layers (see the disadvantages listed above). For this reason, a modeling approach can be very useful, and that is what this work proposes.
In numerical modeling, it is often suitable to avoid a volumetric description of the adhesive layer in order to limit problems that can be involved (e.g., a mesh size that is too small, mesh dependency, too large of a number of degrees of freedom, and too long of a computational time). The classic strategy used for modeling damage in adhesive-bonded joints is based on cohesive zone models (CZMs) [
6], which are described by a traction–separation (TS) law across the cohesive surface. Several TS laws of different shapes (i.e., bilateral, trapezoidal, polynomial) have been proposed (see [
12,
13] and the references therein), and they adequately describe the global response of adhesive-bonded joints [
14,
15,
16,
17]. However, a crucial drawback of CZMs is that they adopt a phenomenological approach, and thus, the model parameters describing the damage/failure behavior of adhesives are not based on their physical properties (e.g., material properties, geometry).
To overcome this drawback, for the past few years, the authors have been working on alternative TS laws, issued by an imperfect interface approach combining continuum damage mechanics and asymptotic homogenization. These imperfect interface laws have already established their effectiveness in taking into account the micromechanical properties of the adhesive, such as anisotropy [
18], micro-cracking, and roughness [
19]. Moreover, they can describe the behavior of
hard adhesive layers (as stiff as adherents) in which both stress and displacement jumps occur [
20,
21,
22]. Recently, the authors provided a new hard imperfect interface model accounting for micro-cracking damage [
23] via an evolution law that is directly related to the mechanical properties of the adhesive.
As a novelty, this paper aims to apply the hard imperfect interface model cited above to the case of adhesive layers in glued butt joints submitted to combined tensile–torsion loads. In detail, a tube-to-tube butt joint configuration is chosen in order to provide an analytical law that, once implemented in a finite element code, can simulate standard characterization tests for structural adhesives.
The presentation of the analytical interface model and its original validation by comparison with experimental data by Murakami et al. [
9] are the subjects of this paper, which is organized as follows: the analytical model is presented in
Section 2; its numerical implementation together with the chosen experimental data from [
9] are detailed in
Section 3; the results are illustrated in
Section 4, and finally, conclusions and perspectives of future work are highlighted in the summary.
2. Analytical Method for Damage Prediction
After introducing the equilibrium problem of the tube-to-tube butt joint, a classical solution is first introduced, corresponding to the perfect contact between the adherents and modeling a very rigid adhesive. Next, a generalization of the classical solution is proposed, taking into account the presence of a very thin deformable adhesive. The latter is described by a model of an imperfect interface proposed in [
22]. Micro-cracking damage within the adhesive is described by using the Kachanov–Sevostianov (KS) model for micro-cracked materials [
24,
25]. Damage evolution is accounted for by the evolution law obtained in [
23] via an asymptotic method.
2.1. Classical Solution for Perfect Contact between the Adherents
The butt-joint specimen is composed of two identical cylindrical adherents that are joined together. The lower basis of the specimen is fixed, and the upper one is subjected to combined tensile force
F and torque
as shown in
Figure 1, where the dimensions of the adherents are also shown. Under the simplifying hypotheses of perfect adhesion, small strains, and linear-elastic material behavior, the stress tensor in the adherents is given by the classical relation
where
is the versor of the
i axis,
the symbol ⊙ is taken to denote the symmetric dyadic product of vectors, and
A and
are the cross-sectional area and the polar moment of inertia, respectively. The stress tensor (
1) is divergence-free, and the surface forces
vanish on the lateral surface of the cylinder, as
is the outward normal to the lateral surface. The resultant vertical force and torque on the upper basis of the cylinder balance the applied force
F and torque
respectively, so equilibrium is ensured.
Assuming the adherents to be made of the same linearly elastic isotropic material with Young’s modulus
Poisson’s ratio
and shear modulus
the homogeneous displacement field in the adherents corresponding to the stress (
1) is
2.2. Generalized Equilibrium Solution for Imperfect Contact between the Adherents
The displacement field (
2) is appropriate for a specimen made of two identical adherents that are perfectly joined. To take into account the presence of a very thin elastic adhesive without describing it geometrically in a numerical model, we propose the original approach to impose an imperfect interface boundary condition that simulates the macroscopic behavior of a very thin elastic adhesive [
20]. Often, structural adhesives have a stiffness that is comparable to the adherents’ stiffnesses; in this case their mechanical behavior cannot be accurately described via a classic spring-like interface model (i.e., the continuity of stresses and discontinuity of displacements), but a hard interface condition also accounting for stress jumps is indicated more. For this reason, we assume the thin adhesive layer to be modeled by the following law of hard imperfect contact proposed in [
22]:
where
is the thickness of the adhesive, and the symbols
and
are taken to denote the jump and the average of the quantity
across the interface separating the two adherents, respectively; the Greek indexes (
) are related to the in-plane (
) quantities; commas denote the first derivatives, and the summation convention is used.
The transmission conditions (
3) and (
4) prescribe jumps in the traction
and displacement
fields across the interface between the two adherents, thus describing the asymptotic behavior of a very thin deformable adhesive made of a general anisotropic linear-elastic material with elasticity coefficients
which are related to the matrices
If the adhesive is modeled as isotropic, with Young’s modulus
and Poisson’s ratio
the matrices
have the form:
To take into account to the presence of the adhesive and enforce the transmission conditions (
3) and (
4), we propose a generalized equilibrium solution, which is obtained by modifying the displacement field (
2) in the upper part of the tube-to-tube butt joint as follows:
where the jumps
possibly dependent on
have to be determined. In the lower part of the tube-to-tube butt joint below the adhesive, for
the displacement field is still given by (
2).
In (
7), the jumps
have to be chosen in order to satisfy the transmission conditions (
3) and (
4). In the presence of the thin deformable adhesive, assuming that the stress field is still given by (
1) and substituting (
1) and (
5)–(
7) into (
3) and (
4), we obtain:
with
where
is the shear modulus of the adhesive.
In view of (
1), (
2), (
7), and (
8), the generalized equilibrium solution is thus characterized by:
The stress field (
1), which is continuous across the adhesive and equilibrated by the applied loads;
A displacement field that is discontinuous across the adhesive, which is given by (
2) below the adhesive (for
) and by (
7) above the adhesive (for
);
A strain field that is continuous across the adhesive, which is given by the symmetric part of the gradient of (
2) (or (
7)).
Notably, the displacement fields above and below the adhesive differ by a rotation in the -plane, given by the jumps and and by a translation along the -axis, given by the jump . The rotation and the translation reproduce the shear and axial deformations, respectively, of a very thin adhesive under the given applied load acting on the tube-to-tube butt joint.
Finally, in the proposed generalized solution that takes the imperfect contact into account, the stress distribution is assumed to be uniform, thus neglecting the effect of stress concentrations on the behavior of the joint. This latter aspect is not addressed in the present paper.
2.3. Micro-Cracking Damaging Adhesive Model
To model a micro-cracking damaging adhesive, we consider the micromechanical homogenization approach proposed by Kachanov and Sevostianov [
24,
25] based on the approximation of non-interacting micro-cracks. The elastic potential in stresses (complementary energy density) of the effective medium yields the following structure for the effective modulus
where
M denotes any shear, Young’s, or bulk moduli:
where
is the modulus of the undamaged matrix or the initial modulus of the adhesive before damage,
is the micro-crack density, thus representing a damage parameter, and the constant
C depends on the particular modulus
M that is considered and on the orientational distribution of defects. For a two-dimensional random distribution of circular voids,
in the Young’s modulus and
in the shear modulus, where
is the Poisson ratio of the undamaged matrix [
24].
2.4. Damage Evolution
Damage evolution is described as an accumulation of micro-cracks by assuming the damage parameter
to increase with time
The evolution of the micro-crack density for the proposed model is described by the following kinetic equation, which was proposed in [
23]:
where
is a positive viscosity parameter, a dot denotes time differentiation,
is a strictly negative parameter,
is the generalized displacement field defined by (
7) above the adhesive and by (
2) below it,
denotes the positive part, and
indicates the component-wise derivative of the stiffness tensor
with respect to
. Note that
also depends on the adhesive layer thickness
.
The kinetic Equation (
13) is a first-order ODE in the unknown damage evolution function
to be solved for the initial condition
. It is important to emphasize that (
13) is directly related to the intrinsic mechanical and damage properties of the adhesive layer. In detail,
is a damage viscosity that influences the velocity of the damage evolution, and
is an energy threshold, which is similar to the energy of adhesion of polymers [
26], after which the damage evolution starts at the adhesive layer.
2.5. Stress–Strain Response
The aim here is to find the stress–strain response of the adhesive in the tube-to-tube butt joint subjected to a combined tensile–torsion loading. The tensile stress
and shear stress
in the adhesive layer are calculated as:
where
R is the outer radius of the joint. The tensile strain
and shear strain
of the adhesive are given by:
respectively, where
is the axial displacement of the adhesive and the square root is the circumferential displacement at the outer diameter of the adhesive. Substituting (
8) into (
16), the normalized tensile stress–tensile strain and shear stress–shear strain are found as follows:
where
are given by (
9) and (
10), respectively. Note that in (
9) and (
10), the moduli
and
depend upon the micro-crack density
through the KS model (cf. (
11)) as follows:
where
is the initial shear modulus of the adhesive. The damage parameter
evolves via the kinetic Equation (
13). By substituting (
2), (
5)–(
7), (
14), and (
18) into (
13) and simplifying, we obtain the following evolution problem for the damage parameter
with
The constants
reported in
Appendix A, depend on the elasticity coefficients of the adherents
on the initial elasticity coefficients of the adhesive
and on the constants
and
Note that tensile and torsion loads are decoupled in (
19). Finally, since we are simulating force-controlled tests, the use of (
15) allows us to eliminate the tensile load
F and the torque
T in favor of the control variables
and
where
and
are the tensile and shear strain rates, respectively.
For pure torsion, i.e., for
and
the evolution problem (
19) admits the simple solution:
where
is the instant at which damage evolution begins:
Substituting (
22) and (
9) into the second of (
17), the shear strain–stress response of the adhesive is obtained:
with
For a pure tensile load, i.e., for
and
or for a combined tensile–torsion loading, a general closed-form solution of the evolution problem (
19) is not available. However, it is possible to obtain a closed-form solution before damage initiation. Indeed, in view of the positivity of the constants
and
in (
20) (cf. the
Appendix A), inspection of (
19) indicates that the instant
of damage initiation for a generic combination of tensile and torsion loads takes the form:
Note that for pure torsion (
and
), (
28) reduces to (
23).
For
the shear stress–strain response is still given by the linear part in (
24), while the tensile stress–strain response takes the following linear form:
where the constants
are given in
Appendix A.
3. Numerical Implementation
The numerical simulations for the pure tensile and for a combined tensile–torsion loading condition were carried out by numerically solving the differential problem (
19) using the commercial software
Mathematica [
27]. For pure torsion loading, the closed-form solution (
24) was used.
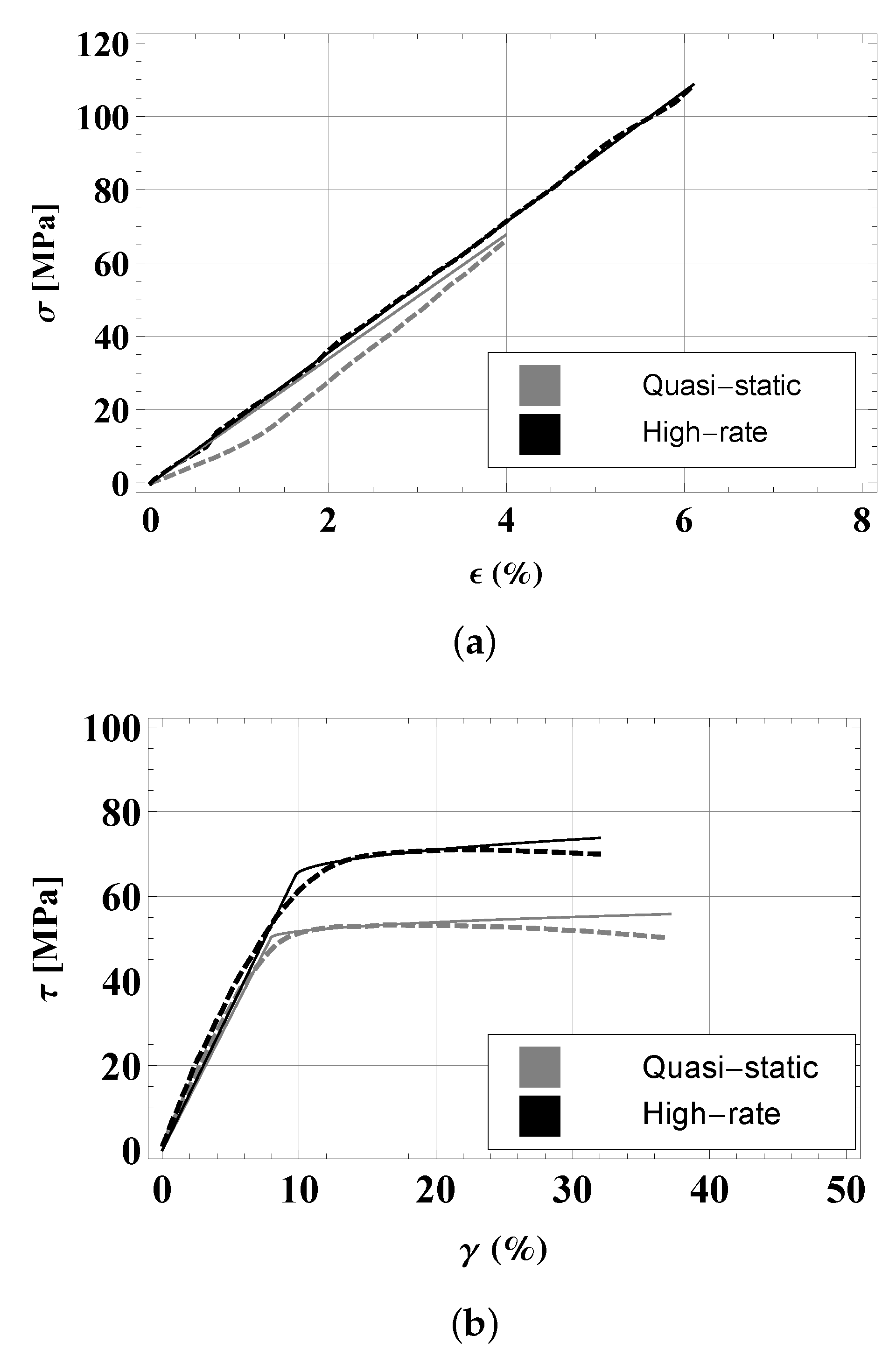
Table 1 and
Table 2 show the geometrical and material parameters of the joint specimen of the experimental study by Murakami and coworkers [
9] that were chosen to compare with those of the proposed model as an original validation. In [
9], the adherents were two S45C carbon steel cylinders joined by a one-component epoxy adhesive (XA7416, 3M Japan Ltd., Tokyo, Japan).
The micromechanical parameters
and
in (
18) were chosen to be equal to
and to
respectively, the latter value being estimated using (
12). The other micromechanical parameters, i.e., the initial value of the damage parameter
the viscosity parameter
and the energy threshold
will be identified in the next subsections starting from the experimental data from [
9].
According to [
9], two different stress rates were considered in the numerical analyses:
MPa/s for the quasi-static (QS) condition and
MPa/s for the high-rate (HR) condition.
For pure tensile and torsion tests, the simulations were stopped at failure, i.e., when the stress reached the tensile and shear limit strengths, respectively. For a combined tensile–torsion test, the tensile and shear stresses were related using a loading angle
:
The tensile and shear strengths estimated experimentally in [
9] for some values of the loading angle are reported in
Table 3.
5. Summary
The behavior of thin adhesive layers in butt joints under combined tensile and torsion loads was modeled by using an imperfect interface approach that merged continuum damage mechanics and asymptotic homogenization. The proposed approach took micro-cracking damage evolution into account, resulting in a ductile stress–strain behavior of the adhesive for the pure torsional tests and in a brittle stress–strain behavior for the pure tensile and combined tensile–torsion tests. In the case of pure torsion (ductile damage behavior), a closed-form solutions was proposed. In the case of brittle damage behavior (pure tensile and combined tensile–torsion loads), a closed-form solution was calculated in the linear stress–strain domain. The comparisons with the experimental data from [
9] gave satisfying results in terms of the failure strains for pure and combined loads in both QS and HR conditions. In all cases, the relative errors between the experimental and simulated failure strains were found to be less than
.
The proposed model has some main limitations. First, stresses in the adhesive layer are supposed to be uniformly distributed. This is not realistic, particularly at the boundaries between the adhesive and adherents, where stress concentrations are known to occur [
11]; therefore, the effect of the stress concentration on tensile strength is not discussed in this paper. Next, for the sake of simplicity, the adhesive thickness was assumed to be constant and uniform in the whole layer, and perfect thickness uniformity is almost impossible to achieve in real applications. Nevertheless, it is possible to easily generalize the analytical model by accounting for a smooth–rough interface (cf. [
19]). Lastly, the viscoplasticity and viscoelasticity that are typical of structural adhesives were not considered in the proposed model. These aspects could be the object of further work.
Despite these limitations, the model is able to accurately reproduce experimental stress–strain behavior for both brittle and ductile damages. Future studies will focus on an experimental protocol for the identification of the model parameters.